Question

A student solving for the acceleration of an object has applied appropriate physics principles and obtained the expression a = a₁ + Fm where a₁ = 3.00 meter/second², F = 12.0 kilogram⋅meter/second² and m =7.00 kilogram. First, which of the following is the correct step for obtaining a common denominator for the two fractions in the expression in solving for a?

a. (m/m times a₁/1) + (1/1 times F/m)
b. (1/m times a₁/1) + (1/m times F/m)
c. (m/m times a₁/1) + (F/F times F/m)
d. (m/m times a₁/1) +(m/m times F/m )

Answer 1

The correct option is option (a).

The acceleration of an object is
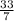`(33)/(7)` m/s².

Step-by-step explanation:

Given expression is

`a=a_1+\frac Fm`

[ divide a whole number by 1 to turn into a fraction. Since
`a_1` is a whole number, so
`a_1` is divided by 1 to turn into a fraction]

`\Rightarrow a=(a_1)/(1)+\frac Fm`

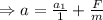
[The l.c.m of the denominators 1 and m is m. Now multiply both numerator and denominator by m of
`\frac{a_1}1` and multiply both numerator and denominator by 1 of
`\frac Fm`]

`\Rightarrow a=(\frac mm*(a_1)/(1))+(\frac 11*\frac Fm)`

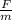The correct option is option (a)

Given that,

F= 12.0 kg.m/s² , m=7.00 kg and
`a_1` = 3.00 m/s²

`\therefore a=(\frac mm*(a_1)/(1))+(\frac 11*\frac Fm)`

`=((7.00)/(7.00)* (3.00)/(1))+(\frac11* (12.0)/(7.00))`

`=(21)/(7)+(12)/(7)`

`=(21+12)/(7)`

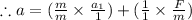
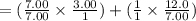
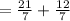
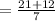
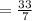`=(33)/(7)` m/s²

The acceleration of an object is
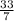`(33)/(7)` m/s².