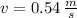Answer:
a)
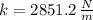 , b)
, b)
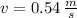
Step-by-step explanation:
a) According to the Principle of Energy Conservation, the kinetic energy of the box is transformed into elastic potential energy.
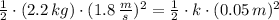
The spring constant is:
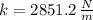
b) The initial speed needed to compress the spring by 1.5 centimeters is: