Answer:
0.07 J
Step-by-step explanation:
Parameters given:
Radius of loop, r = 13 cm = 0.13 m
Change in magnetic field, B = 0.86 T
Time taken, t = 0.67 s
Resistance per unit length of wire, R/L =
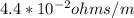
Resistance in the entire length of wire, R = R/L * L (where L = circumference of wire)
R =
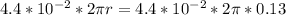
R = 0.036 ohms
Electrical energy is given as the product of Power and Time;
E = P * t
Electrical power, P, is given as:
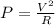
where V = EMF/Voltage
The average EMF induced in a coil is given as:
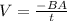
where A = Area of coil =
 =
=
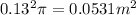
Therefore, Power becomes:
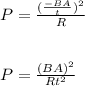
Then, Electrical energy becomes:
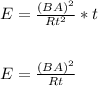
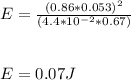
The average electrical energy dissipated in the resistance of the wire is 0.07 J