Question

A 1.103 g sample of sodium fluoride is dissolved in water, and then a precipitate of calcium fluoride is produced by adding a calcium nitrate solution. If the dried calcium fluoride precipitate has a mass of 0.947 g, what is the percent yield?

Answer 1

Answer: The percent yield of the reaction is, 92.0 %

Explanation : Given,

Mass of
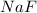`NaF` = 1.103 g

Molar mass of
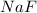`NaF` = 42 g/mol

Molar mass of
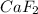`CaF_2` = 78 g/mol

First we have to calculate the moles of
`NaF`

`\text{Moles of }NaF=\frac{\text{Given mass }NaF}{\text{Molar mass }NaF}`

`\text{Moles of }NaF}=(1.103g)/(42g/mol)=0.0263mol`

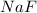
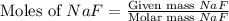
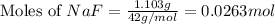
Now we have to calculate the moles of
`CaCl_2`

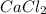
The balanced chemical equation is:

`2NaF+Ca(NO_3)_2\rightarrow CaF_2+2NaNO_3`

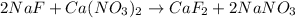
From the reaction, we conclude that

As, 2 mole of
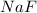`NaF` react to give 1 mole of
`CaF_2`

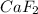
So, 0.0263 mole of
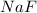`NaF` react to give
`(0.0263)/(2)=0.0132` mole of
`CaF_2`

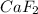
Now we have to calculate the mass of
`CaF_2`

`\text{ Mass of }CaF_2=\text{ Moles of }CaF_2* \text{ Molar mass of }CaF_2`

`\text{ Mass of }CaF_2=(0.0132moles)* (78g/mole)=1.029g`

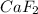
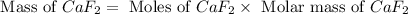
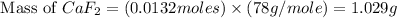
Now we have to calculate the percent yield of the reaction.

`\text{Percent yield}=\frac{\text{Experimental yield}}{\text{Theoretical yield}}* 100`

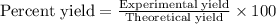
Experimental yield = 0.947 g

Theoretical yield = 1.029 g

Now put all the given values in this formula, we get:

`\text{Percent yield}=(0.947g)/(1.029g)* 100=92.0\%`

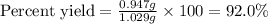
Therefore, the percent yield of the reaction is, 92.0 %