Answer:
The sum of dimensions is 12 in.
Explanation:
Given that, the dimension of the cardboard is 8 in by 8 in.
Let the length of side of the squares which are cut out from cardboard be x
The height of the folded box is = x in
The length of the folded box is =(8-2x) in.
The width of the folded box is = (8-2x) in.
Then the volume of the folded box is= Length × width × height
=(8-2x)(8-2x)x cubic in
=(64x-32x²+4x³) cubic in
Let,
V=64x-32x²+4x³
Differentiating with respect to x
V'= 64 -64x+12x²
Again differentiating with respect to x
V''= -64+24x
For maximum(or for the minimum value)
V'=0
⇒64 -64x+12x²=0
⇒4(3x²-16x+16)=0
⇒3x²-16x+16=0
⇒3x²-12x-4x+16=0
⇒3x(x-4)-4(x-4)=0
⇒(3x-4)(x-4)=0

Now,
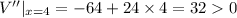
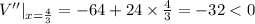
Since at
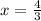 , V''<0, So at
, V''<0, So at
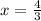 , the volume of the box maximum
, the volume of the box maximum
The height of the folded box is =
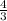 in
in
The length of the folded box is
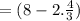
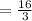 in
in
The width of the folded box is
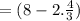
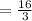 in
in
The sum of dimensions is
=Length+width+height
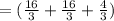 in
in
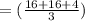 in
in
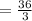 in
in
=12 in