Answer:
The change on the second particle is
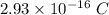 .
.
Step-by-step explanation:
The period of revolution of the particle in the magnetic field is given by the formula as follows :
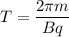
It is given that the magnetic field is uniform. The mass of the second particle is the same as that of a proton but thecharge of this particle is different from that of a proton.
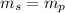
If both particles take the same amount of time to go once around their respective circles. So,
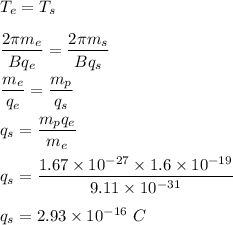
So, the change on the second particle is
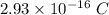 .
.