Question

A horizontal pipe of diameter 0.81 m has a smooth constriction to a section of diameter 0.486 m . The density of oil flowing in the pipe is 821 kg/m3 . If the pressure in the pipe is 7970 N/m2 and in the constricted section is 5977.5 N/m 2 , what is the rate at which oil is flowing

Answer 1

2.06 m³/s

Step-by-step explanation:

diameter of pipe, d = 0.81 m

diameter of constriction, d' = 0.486 m

radius, r = 0.405 m

r' = 0.243 m

density of oil, ρ = 821 kg/m³

Pressure in the pipe, P = 7970 N/m²

Pressure at the constriction, P' = 5977.5 N/m²

Let v and v' is the velocity of fluid in the pipe and at the constriction.

By use of the equation of continuity

A x v = A' x v'

r² x v = r'² x v'

0.405 x 0.405 x v = 0.243 x 0.243 x v'

v = 0.36 v' .... (1)

Use of Bernoulli's theorem

`P+(1)/(2) \rho v^(2)=P' +(1)/(2)\rho'v'^(2)`

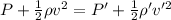
7970 + 0.5 x 821 x 0.36 x 0.36 x v'² = 5977.5 + 0.5 x 821 x v'² from (i)

1992.5 = 357.3 v'²

v' = 5.58 m/s

v = 0.36 x 5.58

v = 2 m/s

Rate of flow = A x v = 3.14 x 0.405 x 0.405 x 2 x 2 = 2.06 m³/s

Thus the rate of flow of volume is 2.06 m³/s.