To solve the problem we will require the concept of Force as a definition of pressure and Area, and the concept of light pressure itself determined by the relationship between intensity and the speed at which light travels. We will match the terms and find the desired force value,
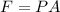
Here,
P = Pressure
A = Area
Pressure due to the light of the sun will be

Here,
I = Intensity
c = Speed velocity
Equation both therms we have that
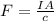
We have a circular area then
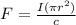
Replacing with our values (Adding the radius of the Earth)

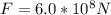
Therefore the Force on Earth due to radiation pressure is
