9514 1404 393
Answer:
Step 4
Explanation:
Step 4 should look like ...
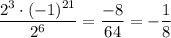
All steps up to that point are correct. Step 4 has the first error.
_____
Comment on the question
It took about 15 minutes to translate the mishmash provided in this problem statement into something sensible. A picture or math symbols are much preferred. At the very least, some line spacing or formatting of the text would be useful.