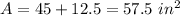Answer:
The area of the trapezoid is 57.5 square inches
Explanation:
we know that
The trapezoid QRST can be divided into a rectangle QRDT and an isosceles right triangle RSD
see the attached figure to better understand the problem
step 1
The area of rectangle is given by the formula
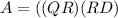
we have
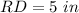 ----> altitude
----> altitude
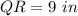
substitute

step 2
Find the area of the isosceles right triangle
The area of triangle is given by the formula
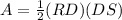
we have
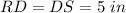 ---> because is an isosceles triangle
---> because is an isosceles triangle
substitute
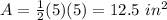
step 3
Adds the areas