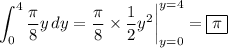Question

Region R is bounded by the curves y = 4x^2 and y = 4. A solid has base R, and cross sections perpendicular to the y-axis are semicircles with the diameter lying in R. The volume of this solid is

A. pi/4
B. pi/2
C. pi
D. 2pi

Answer 1

Each cross section has a diameter equal the horizontal distance between the two "halves" of the parabola. We have

y = 4x² ⇒ x = ±√y/2

so the diameter for some given y would be √y/2 - (-√y/2) = √y.

The volume of a cross section with thickness ∆y is then

π/2 (√y/2)² ∆y = π/8 y ∆y

The total volume of the solid would then be

`\displaystyle \int_0^4 \frac\pi8 y \, dy = \frac\pi8 * \frac12 y^2 \bigg|_(y=0)^(y=4) = \boxed{\pi}`