Simplifying
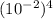 yields
yields
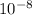 , obtained by multiplying the exponents. This represents a decimal fraction with eight zeros after the decimal point, showcasing the impact of the successive exponentiation. (option A)
, obtained by multiplying the exponents. This represents a decimal fraction with eight zeros after the decimal point, showcasing the impact of the successive exponentiation. (option A)
To simplify
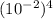 , apply the power of a power rule, which involves multiplying the exponents. In this case, raise the base (10) to the power of the product of the two exponents:
, apply the power of a power rule, which involves multiplying the exponents. In this case, raise the base (10) to the power of the product of the two exponents:
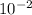 raised to the power of 4 results in
raised to the power of 4 results in
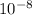 .
.
This simplification follows the principle that when a power is raised to another power, the exponents are multiplied. Therefore,
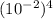 is equivalent to
is equivalent to
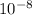 , signifying a decimal fraction with eight zeros after the decimal point.
, signifying a decimal fraction with eight zeros after the decimal point.
So option A is correct.