Answer:
6 inches
Explanation:
To solve this problem, we first need to find the perimeter of the larger rectangle. The formula for a perimeter is:
 . Lets plug in the values:
. Lets plug in the values:
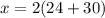

Now that we know that the larger rectangle has a perimeter of 108 and its shorter side is 24 inches, we can cross multiply to find our answer.
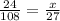
Next we cross multiply. We multiply 108 and x, and we multiply 24 and 27.
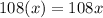
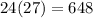
Now, lets put the values above back into the equation:

Our last step is to divide 648 by 108.
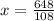
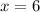
The length of the shorter side of the smaller rectangle is 6 inches.