Question

Let f be the function defined by f(x)=ax^2+bx+2/2x^2−8, where a and b are constants. The graph of f has a horizontal asymptote at y=3, and f has a removable discontinuity at x=2. To make f continues at x=2, f(2) should be defined as what value? Justify your answer

Answer 1

define f(2) = 11/8

Explanation:

To make the graph have a horizontal asymptote at y=3, the coefficient of x^2 in the numerator must be 3 times the coefficient of x^2 in the denominator. That is, a = 3·2 = 6.

In order for there to be a removable discontinuity at x=2, we must have (x-2) a factor of both numerator and denominator. We already know the denominator factors as 2(x-2)(x+2), so we need to find the value of b that makes the numerator have a factor of (x-2).

We can find the required value of "b" using synthetic division. The work shown in the attachment tells us that b=-13 will make division of the numerator by (x-2) have a remainder of zero. So the function is ...

`f(x)=(6x^2-13x+2)/(2(x^2-4))=((6x-1)(x-2))/(2(x+2)(x-2))`

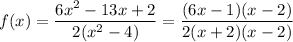
Canceling the factors of (x-2), the simplified function is ...

`f_1(x)=(6x-1)/(2(x+2))\\\\f_1(2)=(6(2)-1)/(2(2+2))=(11)/(8)`

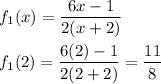
In order to remove the discontinuity at x=2, the function value must be defined as 11/8.