Question

The radius of is circle is 3 kilometers. what is the length of a 180 arc?

Answer 1

The approximate length of the 180-degree arc is 9.428571 kilometers.

How did we get the value?

The length of an arc in a circle is given by the formula:

Arc Length =
`\left( \frac{\text{Angle}}{360} \right) * 2 \pi r`

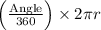
where:

-
`\(\text{Angle}\)` is the central angle in degrees,

-
`\(r\)` is the radius of the circle,

-
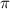`\(\pi\)` is a mathematical constant approximately equal to 3.1428571.

For a 180-degree arc in a circle with a radius of 3 kilometers:

Arc Length =
`\left( (180)/(360) \right) * 2 \pi * 3`

Simplifying this expression:

`\[ \text{Arc Length} = (1)/(2) * 2 \pi * 3 \]`

`\[ \text{Arc Length} = 3 \pi \]`

`\[ \text{Arc Length} \approx 3 * 3.1428571 \approx 9.428571 \, \text{kilometers} \]`

Therefore, the approximate length of the 180-degree arc is 9.428571 kilometers.

Answer 2

9.43km

Explanation:

Length of an arc = 2πr(Ф/360)= 2 x 22/7 x 3 x (180/360)

2 x22/7 x 3 x 1/2 = 22/7 x 3 = 66/7 = 9.42857142857≈9.43