Answer:
The probability that a randomly selected member is a lifetime member, given that he or she bowls regularly is 0.814, option b
Explanation:
Let "M" denotes the event that a club member has a lifetime membership and "B" be denotes the event that the club member bowls.
57% of the members have lifetime membership and bowl regularly. This means, the probability that a randomly selected member has a lifetime membership and bowls regularly =
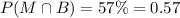
70% of the club members bowl. This means, the probability that a randomly chosen member bowls = P(B) = 70% = 0.70
We have to find the probability that a randomly selected member is a lifetime member, given that he or she bowls regularly. This is conditional probability which can be expressed mathematically as P(M | B)
The formula to calculate the conditional probability is:
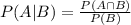
So, the formula for our case will be:
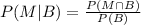
Using the values in this formula we get:
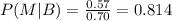
This means, the probability that a randomly selected member is a lifetime member, given that he or she bowls regularly is 0.814