Question

Suppose that y varies inversely as x, and x=9 when y=15. Find the value for k and write the function. Group of answer choices LaTeX: k=\frac{15}{9} k = 15 9 k = 15 9 and LaTeX: y=\frac{9}{15}x y = 9 15 x y = 9 15 x LaTeX: k=\frac{15}{9} k = 15 9 k = 15 9 and LaTeX: y=\frac{15}{9x} y = 15 9 x y = 15 9 x LaTeX: k=135 k = 135 k = 135 and LaTeX: y=\frac{135}{x} y = 135 x y = 135 x LaTeX: k=135 k = 135 k = 135 and LaTeX: y=135x

Answer 1

see explanation

Explanation:

Given that y varies inversely as x then the equation relating them is

y =
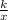`(k)/(x)` ← k is the constant of variation

To find k use the condition that x = 9 when y = 15

15 =
`(k)/(9)` ( multiply both sides by 9 )

k = 135

y =
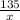`(135)/(x)` ← equation of variation