Given:
The given expression is

We need to determine the simplified value of the given expression.
Simplification:
Since, the given expression is in the form of
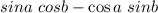 , the given expression can be simplified using the identity
, the given expression can be simplified using the identity
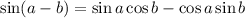
Comparing the given expression with the identity, we get;
 and
and

Using this in the identity, we get;

Simplifying, we get;

Thus, the simplified value of the given expression is

Hence, Option c is the correct answer.