Given:
Rate of change =

Graph passes through the point (4, 10.5)
To find:
The equation of the function of the graph.
Solution:
Rate of change is same as the slope
Slope (m) =

Here
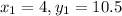
Using point-slope formula:

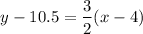

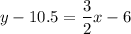
Add 10.5 on both sides.
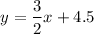
The equation of the function is
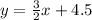 .
.