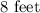Question

A pool measuring 12 meters by 30 meters is surrounded by a path of uniform width. If the area of the pool and path combined is 1288 square meters, what is the width of the path?

Answer 1

`8\text{ feet}`

Explanation:

GIVEN: A pool measuring
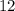`12` meters by
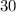`30` meters is surrounded by a path of uniform width. If the area of the pool and path combined is
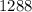`1288` square meters.

TO FIND: Width of the path.

SOLUTION:

Consider the figure attached.

let the width of the path be
`=x`

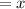
Total width of pool including pool
`=12+2x`

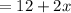
Total length of pool including pool
`=30+2x`

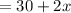
Now,

Area of pool and path combined
`=1288\text{ sq m}`

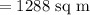
area of pool and path combined
`=(\text{length})*\text{width}`

`=(30+2x)*(12+2x)`

`(30+2x)*(12+2x)=1288`

`{x}^2+21x-232=0`

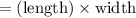
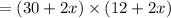
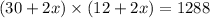
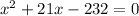
solving equation we get
`x=8\text{ feet}`

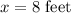
Hence the width of the path is
`8\text{ feet}`