Rate of interest is 12.232%, if Nakiri invests $8,000 at age 18 and she hopes the investment will be worth $64,000 when she turns 35.
Explanation:
The given is,
Investment amount $8000 at age of 18
Future worth $64000 at age of 35
Step:1
Formula to calculate the future amount with an interest of compounded continuously,
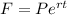 ..........................(1)
..........................(1)
Where,
F - Future amount
P - Present amount
r - Rate of interest
t - No.of years
Constant, e = 2.7183
From given,
P = $8000
F = $64000
t = 35-18 = 17 years
Equation (1) becomes,

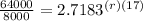
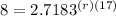
Take log on both sides,
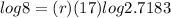
Substitute log values of 8 and 2.7183
0.90308999 = ( r × 17 ) (0.4342974)
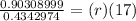
2.07942 = ( r × 17 )
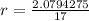
r = 12.232
Result:
Rate of interest is 12.232%, if Nakiri invests $8,000 at age 18 and she hopes the investment will be worth $64,000 when she turns 35.