Answer:
Area = 748

which coincides with the first answer shown in the list of possible options.
Explanation:
Notice that the area of the figure is equivalent to finding the area of 4 right angle triangles and adding them. Please refer to the attached image to follow the explanation.
In the attached figure you can see printed in red the height "H" of the two right angle triangles two the right of the image. Both with the same base length (17 mm). On the left hand side of the image, you see typed in blue the height "h" of the two right angle triangles that also have base 17 mm shared with the counterparts of the right.
The addition of these triangles areas would be the area of the total figure, and based on the formula for the area of a triangle "base x height/2", the addition of these 4 areas becomes:
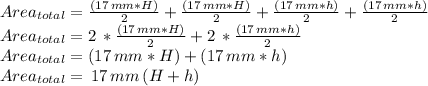
where we have extracted the common factor 17 mm from both terms in the last two steps, leaving a quantity in parenthesis "H + h", which is exactly the dimension 44 mm shown in the picture as the addition of the two heights of the triangles opposite by their bases.
Therefore, the area of the figure is the product of 17 mm times 44 mm = 748
