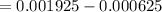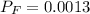Question

(a) A lamp has two bulbs of a type with an average lifetime of 1600 hours. Assuming that we can model the probability of failure of these bulbs by an exponential density function with mean μ = 1600, find the probability that both of the lamp's bulbs fail within 1800 hours. (Round your answer to four decimal places.)(b) Another lamp has just one bulb of the same type as in part (a). If one bulb burns out and is replaced by a bulb of the same type, find the probability that the two bulbs fail within a total of 1800 hours. (Round your answer to four decimal places.)

Answer 1

Complete Question

The complete question is shown on the first uploaded image

Answer:

a

The probability is
`P_T= 0.4560`

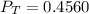
b

The probability is
`P_F= 0.0013`

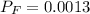
Explanation:

From the question we are told that

The mean for the exponential density function of bulbs failure is
`\mu = 1600 \ hours`

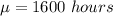
Generally the cumulative distribution for exponential distribution is mathematically represented as

`1 - e^(- \lambda x)`

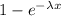
The objective is to obtain the p=probability of the bulbs failure within 1800 hours

So for the first bulb the probability will be

`P_1(x < 1800)`

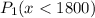
And for the second bulb the probability will be

`P_2 (x< 1800)`

So from our probability that we are to determine the area to the left of 1800 on the distribution curve

Now the rate parameter
`\lambda` is mathematically represented as

`\lambda`
`= (1)/(\mu)`

`\lambda = (1)/(1600)`

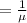
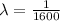
The probability of the first bulb failing with 1800 hours is mathematically evaluated as

`P_1(x < 1800) = 1 - e^{(1)/(1600) * 1800 }`

`= 0.6753`

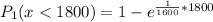
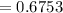
Now the probability of both bulbs failing would be

`P_T=P_1(x < 1800) * P_2(x < 1800)`

`= 0.6375 * 06375`

`P_T= 0.4560`

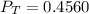
Let assume that one bulb failed at time
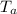`T_a` and the second bulb failed at time
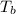`T_b` then

`T_a + T_b = 1800\ hours`

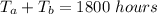
The mathematical expression to obtain the probability that the first bulb failed within between zero and
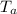`T_a` and the second bulb failed between
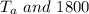`T_a \ and \ 1800` is represented as

`P_F=\int_(0)^(1800)\int_(0)^(1800-x) \f{\lambda }^(2)e^(-\lambda x)* e^(-\lambda y)dx dy`

`=\int_(0)^(1800) {\lambda }e^(-\lambda x)\int_(0)^(1800-x) {\lambda } e^(-\lambda y)dx dy`

`=\int_(0)^(1800) {(1)/(1600) }e^(-\lambda x)\int_(0)^(1800-x) (1)/(1600 ) e^(-\lambda y)dx dy`

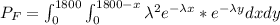
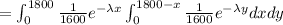
`=\int_(0)^(1800) {(1)/(1600) }e^(-\lambda x)[e^(- \lambda y)]\left {1800-x} \atop {0}} \right. dx`

`=\int_(0)^(1800) {(1)/(1600) }e^{-(x)/(1600) }[e^{- (1800 -x)/(1600) }-1] dx`

`=[ {(1)/(1600) }e^{-(1800)/(1600) }-(1)/(1600)[e^{- (x)/(1600) }] \left {1800} \atop {0}} \right.`

`=[ {(1)/(1600) }e^{-(1800)/(1600) }-(1)/(1600)[e^{- (1800)/(1600) }] -[[ {(1)/(1600) }e^{-(1800)/(1600) }-(1)/(1600)[e^(-0)]`

`=[(1)/(1600) e^{-(1800)/(1600) } - (1)/(1600) e^(-0) ]`

`=0.001925 -0.000625`

`P_F= 0.0013`

Answer 2

a) 0.4560

Explanation:

a) Failure of Bulbs has an Exponential Density Function with a mean(\mu) = 1600 hours

Cumulative distribution function of Exponential Distribution is given by: 1-e^{-\λ *x}

We want the probability of both bulbs failing before 1800 hours

This means P_{1st bulb} (X<1800) and P_{2nd bulb} (X<1800) =??

So, we want area to the left of value 1800 on the distribution curve

Here, λ = 1 / 600

P(X<1800) = 1-e^{-λ *x}

=1-e^-{ 1 / 1600 *1800}

=0.6753

This is the probability that one bulb will fail within 1800 hours.

Probability of both bulbs is simply, 0.6753 * 0.6753 = 0.4560