Question

You have been asked by the Pharmacist to prepare 3 liters of a 30% strength solution. Your pharmacy stocks the active ingredient in 8 oz bottles of 70% strength. How many bottles of the active ingredient will you need to open to complete this request?

A. 14
B. 10
C. 6
D. 3

Answer 1

C. 6

Explanation:

Let x liters represent 70% of the solution.

Let us assume that solution is diluted with water, which has a 0% strength.

We have been given that you have been asked by the Pharmacist to prepare 3 liters of a 30% strength solution. Your pharmacy stocks the active ingredient in 8 oz bottles of 70% strength.

The amount of water would be 3 liters minus liters of 70% solution that is
`3-x`.

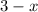Now we can set an equation where 70% of x plus 0% of
`(3-x)` is equal to 30% of 3 liters.

`70\%\cdot x+0\%(x-3)=30\%\cdot 3`

`(70)/(100)\cdot x+(0)/(100)(x-3)=(30)/(100)\cdot 3`

`0.70\cdot x+0=0.30\cdot 3`

`0.70x=0.90`

`(0.70x)/(0.70)=(0.90)/(0.70)`

`x=1.2857`

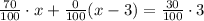
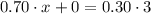
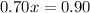
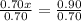
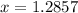
Since our amount is in liters, so we need to convert 1.2857 liters into oz.

1 liter = 33.814 oz

1.2857 liters = 1.2857*33.814 oz = 43.4746598 oz

Since each bottle contains 0 oz, so we will divide 43.4746598 by 8 to find the number of bottles.

`\text{Required number of bottles}=(43.4746598)/(8)`

`\text{Required number of bottles}=5.43433`

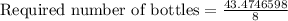
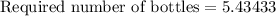
Since we cannot open 0.43433 of a bottle, so we need to open 6 bottles and option C is the correct choice.