Answer:
45.72°
Step-by-step explanation:
A ray of light traveling in water is incident at a water-air interface.
Let the angle of incidence be = i which is relative to a right angle
refractive index of water
 = 1.333
= 1.333
refractive index

By applying snell's law of refraction
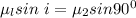
1.33 sin i = 1 (1)
sin i =
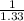
sin i = 0.7159
i = sin ⁻¹(0.7159)
i = 45.72°
Thus, If the refractive index of water is 1.333, then the angle of incidence must have been 45.72°