Question

The following picture is a square pyramid where DE=8 cm and m∠ADE=60°.

Find the surface area of the pyramid in both exact form and approximate form.
Round your approximate answer to one decimal place.
(Make sure you include formulas and numbers in the formulas as part of your solution.
Also include units in your final answers.)

Answer 1

174.9 cm²

Explanation:

AD = 2 × (8cos60)

AD = 8

Base = 8²

= 64

4 triangles = 4(½ × 8 × 8sin60)

= 64sqrt(3)

SA = 64 + 64sqrt(3)

174.8512517

Answer 2

Given:

The length of DE is 8 cm and the measure of ∠ADE is 60°.

We need to determine the surface area of the pyramid.

Length of AD:

The length of AD is given by

`cos 60^(\circ)=(FD)/(8)`

`4=FD`

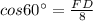
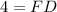
Length of AD = 8 cm

Slant height:

The slant height EF can be determined using the trigonometric ratio.

Thus, we have;

`sin \ 60^(\circ)=(EF)/(8)`

`sin \ 60^(\circ) * 8=EF`

`(√(3))/(2) * 8=EF`

`4√(3)=EF`

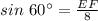
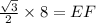
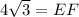
Thus, the slant height EF is 4√3

Surface area of the square pyramid:

The surface area of the square pyramid can be determined using the formula,

`SA=Area \ of \ square + (1)/(2) (Perimeter \ of \ base ) (slant \ height)`

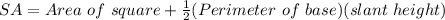
Substituting the values, we have;

`SA=8^2+(1)/(2)(8+8+8+8)(4 √(3))`

`SA=64+(1)/(2)(32)(4 √(3))`

`SA=64+(16)(4 √(3))`

`SA=64+64 √(3)`

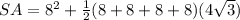
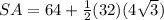
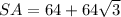
The exact form of the area of the square pyramid is
`64+64 √(3)`

Substituting √3 = 1.732 in the above expression, we have;

`SA = 64 + 110.848`

`SA = 174.848`

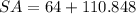
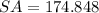
Rounding off to one decimal place, we get;

`SA = 174.8`

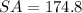
Thus, the area of the square pyramid is 174.8 cm²