Answer:
-Exponential Decay
-Decay factor is (1-0.05)
Explanation:
-Given that the number decreases by a defined rate each year from the initial size by 5%,
-This is an exponential decay function of the form:
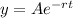
Where:
 is the quantity/size after time t
is the quantity/size after time t
 is the initial size
is the initial size
 is the rate of decay
is the rate of decay
-Our function can the be written as
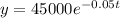
Hence, the decay rate/factor is 0.05
#Alternatively
The exponential decay can be of the form:
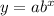
Where:
y is the size at time x, a is the initial size, x is time and b is the decay factor.
b is of the form
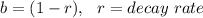
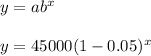
Hence, the decay factor is (1-0.05)