Question

Given f(x)=10x over 2+7, find f^-1(x)

I have provided a picture of the equation for it to be easier to read

Answer 1

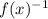`f(x)^(-1)` = 1/(5x+7) [ 1 over (5x + 7)] with x≠ -1.4

Explanation:

Given the function f(x), if f(x) ≠ 0, we would have the formula as following:

+)
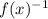`f(x)^(-1)` = 1/f(x)

We have the given equation:

f(x) = (10x/2) + 7 = 5x + 7

f(x) ≠ 0 when and only when (5x + 7) ≠ 0

(5x + 7) ≠ 0

⇔ 5x ≠ 0 - 7

⇔ 5x ≠ -7

⇔ x ≠ -7 ÷ 5

⇔ x ≠ -1.4

So with x≠ -1.4, f(x) ≠ 0, we have:

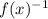`f(x)^(-1)` = 1/f(x) = 1/ (5x + 7)

Conclusion:
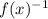`f(x)^(-1)` = 1/(5x+7) [ 1 over (5x + 7)] with x≠ -1.4