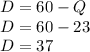Question

Gabe has 60 coins consisting of quarters and dimes. The combined value of the coins is $9.45. How many quarters and dimes does Gabe have?

Answer 1

There are 23 quarters and 37 dimes in the collection

Explanation:

Let's name the two unknowns we need to find :

Q = number of quarters in the collection

D = number of dimes in the collection

So our first equation on the number of coins we have in the collection can be written as:

Q + D = 60

Now let's write an equation that takes care of the total value of the collection ($ 9.45), having in mind that each quarter is worth $ 0.25, and each dime is $ 0.10:

`Q\,0.25+D\,0.10 = 9.45`

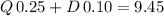
So let's solve this system of linear equations in "Q" and "D" by substitution. We use the first equation to solve for "d" for example, and then use this to substitute for D in the second equation:

`D=60-Q\\\\0.25\,Q+0.10\,D = 9.45\\0.25\,Q+0.10\,(60-Q) = 9.45\\0.25\,Q+6-0.1Q=9.45\\0.25\, Q-0.1\,Q=9.45-6\\0.15\,Q=3.45\\Q=(3.45)/(0.15) \\\\Q=23`

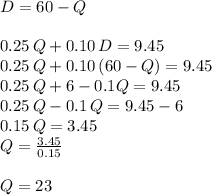
Then, there are 23 quarters in the collection. We can find the number of dimes via the substitution equation we used:

`D=60-Q\\D=60-23\\D=37`