Given:
The composite figure consists of two semicircle and a square.
The length of the sides of the square is 2 inches.
The diameter of the semicircle is 2 inches.
We need to determine the area of the composite figure.
Area of the square:
The area of the square can be determined using the formula,
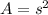
Substituting s = 2, we get;
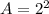
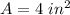
Thus, the area of the square is 4 in²
Area of the semicircle:
The area of the semicircle can be determined using the formula,
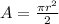

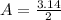
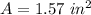
Thus, the area of the semicircle is 1.57 in²
Area of the composite figure:
The area of the composite figure can be determined by adding the area of the square and the area of the two semicircles.
Thus, we have;
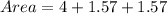
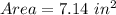
Thus, the area of the composite figure is 7.14 in²
Hence, Option c is the correct answer.