Question

How many cubes with side lengths of 1/4cm does it take to fill the prism?

Length: 2cm
Width: 7/4cm
Height: 1cm

Answer 1

Given:

The length of the rectangular prism is 2 cm.

The width of the prism is
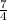`(7)/(4)` cm.

The height of the prism is 1 cm.

The side lengths of the cube is
`(1)/(4)` cm.

We need to determine the number of cubes with side lengths
`(1)/(4)` would fill the prism.

Volume of the cube:

The volume of the cube can be determined using the formula,

`V=s^3`

Substituting the value, we get;

`V=((1)/(4))^3`

`V=(1)/(64)`

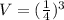
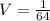
Thus, the volume of the cube is
`(1)/(64) \ cm^3`

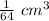
Volume of the rectangular prism:

Volume of the rectangular prism can be determined using the formula,

`V=lwh`

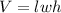
Substituting the values, we get;

`V=2* (7)/(4) * 1`

`V=3.5 \ cm^3`

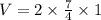
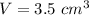
Thus, the volume of the rectangular prism is 3.5 cm³

Number of cubes:

The number of cubes can be determined by dividing the volume of the prism by the volume of the cube.

Thus, we have;

`No. \ of \ cubes=(3.5)/((1)/(64))`

`No. \ of \ cubes=3.5 * 64`

`No. \ of \ cubes=224`

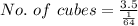
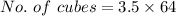
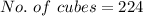
Thus, the number of cubes that fill the prism are 224 cubes.