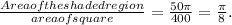Question

PLEASE I NEED HELP WITH MY MATHS HOMEWORK< I HAVE SO MUCH HOMEWORK DURING THIS QUARANTINE

USE THE IMAGE ATTACHED BELOW TO HELP ME
The diagram shows a square ABCD with sides of length 20cm
It also shows a semicircle and arc of a circle.
AB is the diameter of the semicircle
BD is an arc of a circle with centre A

a) Find the area of the shaded region giving your answer in terms of π
You must show your working,clearly stating the area of the semicircle

Answer 1

Explanation:

Answer 2

a) The area of the shaded region is 50π.

b)
`(Area of the shaded region)/(area of square) = (\pi)/(8) .`

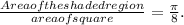
Explanation:

Step 1:

The area of a circle
`= \pi r^(2).`

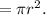
The area of a semi-circle
`= (\pi r^(2) )/(2) .`

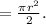
The area of a quarter-circle
`= (\pi r^(2) )/(4) .`

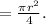
The shaded region's area is obtained by subtracting the area of the semi-circle with a radius of 10 cm from the area of a quarter-circle with a radius of 20 cm.

The area of the semi-circle with a radius of 10 cm
`= (\pi (10^(2)) )/(2) .`

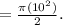
The area of the quarter-circle with a radius of 20 cm
`= (\pi (20^(2)) )/(4) .`

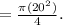
Step 2:

The area of the shaded region is obtained by subtracting these areas.

The area of the shaded region
`= (\pi (20^(2)) )/(4) - (\pi (10^(2)) )/(2) .`

`(\pi (20^(2)) )/(4) - (\pi (10^(2)) )/(2) = (\pi)/(2) ((20^(2) )/(2) -10^(2) ).`

`(\pi)/(2) ((20^(2) )/(2) -10^(2) ) = (\pi)/(2) ((400)/(2) -100 ) = (\pi)/(2) (100).`

`(\pi)/(2) (100) = 50 \pi.`

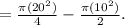
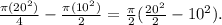
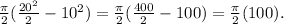
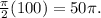
The area of the shaded region is 50π square units.

Step 3:

The area of a square is the square of the side length.

The area of the square
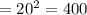`= 20^(2) = 400` square cm.

b)
`(Area of the shaded region)/(area of square) =(50\pi)/(400) =(\pi)/(8) .`