Question

Solve for the missing side. round to the nearest 10th.

Answer 1

• 6.7 mm

`\:`

Explanation :

• This is Right Angled Trian1gle.

`\:`

Solution :

• We'll solve this using the Pythagorean Theorem.

`\:`

Where,

• VW (9 mm) is the Hypotenuse.

• UV (6 mm) is the Base.

• UW is the Perpendicular .

`\:`

We know that,

`{\longrightarrow \pmb{\mathbb {\qquad (UV) {}^(2) + (UW) {}^(2) =( VW) {}^(2) }}} \\ \\`

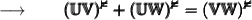
Now, we will substitute the given values in the formula :

`{\longrightarrow \pmb{\sf {\qquad (6) {}^(2) + (UW) {}^(2) =( 9) {}^(2) }}} \\ \\`

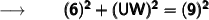
We know that, (6)² = 36 and (9)² = 81. So,

`{\longrightarrow \pmb{\sf {\qquad 36 + (UW) {}^(2) =81}}} \\ \\`

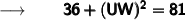
Now, transposing 36 to the other side we get :

`{\longrightarrow \pmb{\sf {\qquad (UW) {}^(2) =81 - 36 }}} \\`

`{\longrightarrow \pmb{\sf {\qquad (UW) {}^(2) = 45}}} \\ \\`

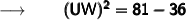
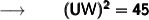
Now, we'll take the square root of both sides to remove the square from UW :

`{\longrightarrow \pmb{\sf {\qquad \sqrt{(UW) {}^(2)} = √( 45)}}} \\ \\`

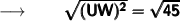
When we take the square root of (UW)² , it becomes UW,

`{\longrightarrow \pmb{\sf {\qquad UW = √(45)}}} \\ \\`

We know that, square root of 45 is 6.708.

`{\longrightarrow \pmb{\sf {\qquad UW \approx6.708}}} \\ \\`

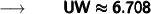
So,

• The measure of the missing side (UW) is 6.7 mm (Rounded to nearest tenth)