Answer:
Null Hypothesis:
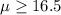
Alternative hypothesis:
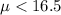
And the correct option for this case would be:
H0:μ=16.5; Ha:μ<16.5, which is a left-tailed test
And the data for this case we have this sample data:
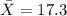 the sample mean
the sample mean
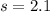 the sample deviation
the sample deviation
n =20 the sample size
Explanation:
Previous concepts
The null hypothesis attempts "to show that no variation exists between variables or that a single variable is no different than its mean"
The alternative hypothesis "is the hypothesis used in hypothesis testing that is contrary to the null hypothesis"
Solution to the problem
For this case we want to test if the mean amount of time American teenagers watch social media posts per month is greater than the mean amount of time last year (alternative hypothesis), and the system of hypothesis are:
Null Hypothesis:
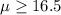
Alternative hypothesis:
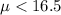
And the correct option for this case would be:
H0:μ=16.5; Ha:μ<16.5, which is a left-tailed test
And the data for this case we have this sample data:
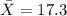 the sample mean
the sample mean
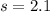 the sample deviation
the sample deviation
n =20 the sample size