Question

Marta invests $8500 in a new savings account which earns 5.0% annual interest, compounded continuously. What will be the value of her investment after 5 years?

Answer 1

The value of her investment after 5 years is $10,914.22

What will be the value of her investment after 5 years?

Accrued value = A

Principal, P = $8500

Interest rate, r = 5% = 0.05

Time, t = 5 years

`A = {Pe}^(rt)`

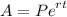
Where,

e is a constant = 2.61828

So,

`A = {Pe}^(rt)`

`A = {8,500.00(2.71828)}^((0.05 * 5))`

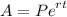
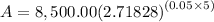
Therefore,

A = $10,914.22

Answer 2

Given:

Investment = $8500

Rate of interest = 5.0%

Time = 5 years

Compounded continuously

To find:

The value of investment after 5 years.

Solution:

Rate = 5.0%

`=(5)/(100)`

Rate = 0.05

Compound interest formula if interest compounded continuously:

`A = P e^(rt)`

where P is the investment and r is the rate and t is the time.

`A = 8500 * e^(0.05 * 5)`

`A = 8500 * e^(0.25)`

The value of
`e^(0.25)=1.284025`.

`A = 8500 * 1.284025`

`A=10914.21`

The value of the investment after 5 years is $10914.21.