Question

How do you add vectors? Add the vector <3,4> to the vector that goes 7 units at an angle of 2π/3.

Answer 1

The sum of the two vectors is the vector <-0.5 , 4+3.5
`√(3)` >

Explanation:

The horizontal component (x) of a vector whose magnitude is b units and its direction is Ф° is b cos Ф

The vertical component (y) of a vector whose magnitude is b and its direction is Ф is b sin Ф

The vector is <b cos Ф , b sin Ф>

∵ The vector goes 7 units at an angle
`(2\pi )/(3)`

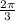
- That means its magnitude is 7 and its direction is
`(2\pi )/(3)`

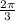
∴ x = 7 cos(
`(2\pi )/(3)` )

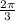∴ y = 7 sin(
`(2\pi )/(3)` )

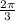∵ cos(
`(2\pi )/(3)` ) =
`-(1)/(2)`

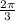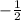
∵ sin(
`(2\pi )/(3)` ) =
`(√(3))/(2)`

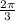- Substitute them in x and y

∴ x = (7)(
`-(1)/(2)` )

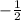∴ x = -3.5

∴ y = (7)(
`(√(3))/(2)` )

∴ y = 3.5
`√(3)`

∴ The vector is <-3.5 , 3.5
`√(3)`>

Now lets add the vectors by adding xs and ys components

∵ <3 , 4> + <-3.5 , 3.5
`√(3)` > = <3 + -3.5 , 4 + 3.5
`√(3)` >

∴ <3 , 4> + <-3.5 , 3.5
`√(3)`> = <-0.5 , 4+3.5
`√(3)` >

∴ The sum of the two vectors is the vector <-0.5 , 4+3.5
`√(3)` >