Answer:

Explanation:
The missing part is: "A cylinder and 2 half spheres. All have a radius of 6 millimeters. The cylinder has a height of 10 millimeters."
You need to use the following formulas to solve the exercise:
1. The volume of a cylinder can be calculated with:
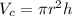
Where "r" is the radius and "h" is the height.
2. The volume of a sphere can be calculated with:
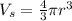 Where "r" is the radius.
Where "r" is the radius.
In this case you know that the cylinder and the sphere have a radius of 6 millimeters and the height of cylinder is 10 millimeters. Then, you can substitute values into each formula in order to find the volumes:
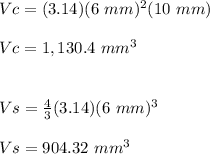
Adding them, you get that the volume of the composite figure is:
