Answer:
A) The circular one
Step-by-step explanation:
When there is a loop of wire in a magnetic field, and the magnetic flux through the coil changes, an electromotive force is induced in the coil, according to Faraday-Newmann-Lenz law:
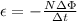
where
N is the number of turns in the coil
 is the change in magnetic flux through the coil
is the change in magnetic flux through the coil
 is the time interval
is the time interval
The variation of flux through a coil can be written as
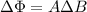
where
A is the area of the coil
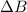 is the change in magnetic field
is the change in magnetic field
Here we have three loops of wire: one circular, one rectangular, one square.
The length of the wire used for the 3 loops is the same, therefore their perimeter is also the same.
The change in magnetic flux is directly proportional to the area enclosed by the loop: therefore, the loop that will experience the greatest induced emf is the one having the greatest area.
Since the circle is the 2D figure that maximizes the area for a given perimeter, this means that the circular loop has the greatest area, and so also the greatest induced emf.