Question

The probabilities and the number of automobiles lined up at a Lakeside Olds at opening time (7:30 a.m.) for service arecol1 Number 1 2 3 4col2 Probability 0.40 0.03 0.06 0.51On a typical day, how many automobiles should Lakeside Olds expect to be lined up at opening?

Answer 1

EX=2.68 ≈ 3

Lakeside Olds should expect 3 automobiles lined up at opening

Explanation:

Expected Value of a Discrete Probability Distribution

Given a discrete probability distribution of values

x={x1,x2,x3...,xn}

And probabilities

p={p1,p2,p3,...,pn}

Provided the sum of all probabilities is 1, then the expected value of the distribution is

EX =
`\sum x_ip_i`

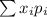
The data refers to the number of automobiles lined up at Lakeside Olds at opening time for service:

x={1,2,3,4}

And probabilities

p={ 0.40 , 0.03 , 0.06 , 0.51 }

Checking the sum: 0.40 + 0.03 + 0.06 + 0.51 = 1

Now compute the expected value

EX= 1*0.40+2*0.03+3*0.06+4*0.51

EX=2.68 ≈ 3

Lakeside Olds should expect 3 automobiles lined up at opening