Question

Aisha is sitting on frictionless ice and holding two heavy ski boots. Aisha weighs 637 N, and each boot has a mass of 4.50 kg. Aisha throws both boots forward at the same time, at a velocity of 6.00 m/s relative to her. What is Aisha's resulting velocity?

Answer 1

-0.73 m/s

Step-by-step explanation:

We can solve this problem by using the law of conservation of momentum.

In fact, in absence of external forces (the ice is frictionless, so no friction), the total momentum of Aisha + two boots is conserved.

At the beginning, their total momentum is zero, since they are at rest:

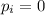`p_i = 0` (1)

After, their total momentum is:

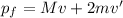`p_f = Mv + 2mv'` (2)

where:

M is Aisha's mass

v is Aisha's velocity relative to the ground

m = 4.50 kg is the mass of each boot

v' is the boot's velocity relative to the ground

We can find:

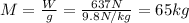`M=(W)/(g)=(637 N)/(9.8 N/kg)=65 kg` is Aisha's mass (where W = 637 N was her weight)

v' can be rewritten as:

`v'=v+6`

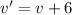
because 6 m/s is the velocity of the boots relative to her, while v' is their velocity relative to the ground.

Substituting and combining (1) and (2) we find:

`0=Mv+2m(v+6)\\0=Mv+2mv+12m\\v=(-12m)/(M+2m)=(-12(4.50))/(65+2(4.50))=-0.73 m/s`

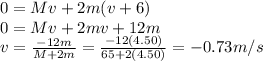
and the negative sign indicates that the direction is opposite to that of the boots.