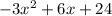Question

write the equation in standard form of the function with x intercepts at (4,0) and (-2,0) that has been reflected across the x axis with a vertical stretch of 3

Answer 1

`-3x^(2) +6x + 24`

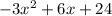
Step-by-step explanation

since there are 2 x-intercepts, the lowest degree the function could possibly have is 2, so this could be the graph of a parabola

since our zeros are at x = 4 and x = -2, our factors are (x - 4) and (x + 2)

our equation so far is

(x - 4)(x + 2)

=
`x^2 -4x + 2x - 8`

=
`x^(2) -2x - 8`

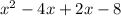
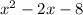
since the function is reflected across the x-axis, multiply the equation by -1

`-(x^(2) -2x - 8)`

=
`-x^(2) +2x + 8`

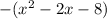
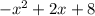
since the function has a vertical stretch of 3, multiply the equation by 3

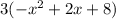
`3(-x^(2) +2x + 8)`

=
`-3x^(2) +6x + 24`