Given:
m∠D = 5x - 10
m(ar EC) = 60°
m(ar RB) = 13x + 7
To find:
The value of x.
Solution:
If two secants intersect outside a circle, then the measure of the angle formed is one-half the positive difference of the measures of the intercepted arcs.

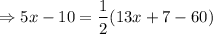
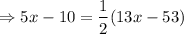
Multiply by 2 on both sides.
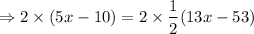

Add 53 on both sides, we get
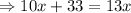
Subtract 10x on both sides, we get
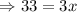
Divide by 3 on both sides, we get

The value of x is 11.