Answer:
Therefore the required probability is 0.83427.
Explanation:
Binomial (n,p) distribution:
A discrete random variable X having the set {0,1,2,3.....,n} as the spectrum, is said to have binomial distribution with parameter n=the number of trial in the binomial experiment,p= the probability of success on an individual trial, if the probability mass function of X is given by
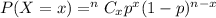 for x= 0,1,2,....
for x= 0,1,2,....
=0 elsewhere
where n is a positive integer and 0<p<1.
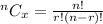
Given that,
n= 9 and p=0.25
We are interested to find P(x<4).
P(x<4)
=P(x=0)+P(x=1)P(x=2)+P(x=3)
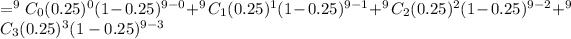
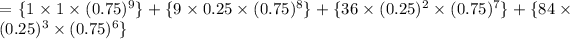
≈ 0.83427
Therefore the required probability is 0.83427.