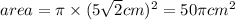Question

The area of the square is 100 square centimeters. What is the area of the circle?

Answer 1

• Since the question is incomplete, see the figure attached and the explanation below.

Step-by-step explanation:

Since the figure is missing, I enclose the figure of a square inscribed in a circle.

Since the area of a square is the side length squared, you can determine the side length:

• Area = (side length)²

• 100 cm² = (side lenght)²

`side\text{ }length=√(100cm^2)=10cm`

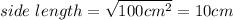
From the side length, you can find the diagonal of the square, which is equal to the diameter of the circle, using the Pythagorean theorem:

• diagonal² = (10cm)² + (10cm)² = 2 × (10cm)²

`diagonal=√(2* (10cm)^2)\\ \\ diagonal=10√(2) cm`

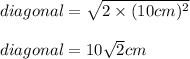
The area of the circle is π (radius)².

• radius = diameter/2 = diagonal/2

`area= \pi * (5√(2)cm)^2=50\pi cm^2`