Answer:
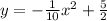
Explanation:
We want to prove algebraically that:

is a parabola.
We use the relations
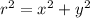
and
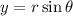
Before we substitute, let us rewrite the equation to get:
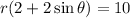
Or
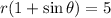
Expand :
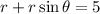
We now substitute to get:

This means that:

Square:
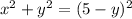
Expand:
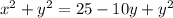

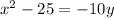

This is a parabola (0,2.5) and turns upside down.