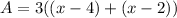Answer:
C. 3((x+4) + (x-2))
Explanation:
Area of a triangle:

Area of the left triangle:
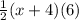
The 6 multiplied by the 1/2 equals 3, so:
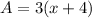
Area of the right triangle:
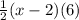
Again, the 6 multiplied by the 1/2 equals 3, so:
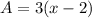
Adding the two areas together equals:
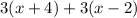
We can factor out the 3 from both, giving us the final answer: