Answer:
a) 12.55ft/s
b) N = 5.392lb
Step-by-step explanation:
Image to question is attached
•Displacement of spring at A
óa = (10+12)-12 = 12in
• displacement of spring at C
óc =
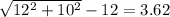
a)for potential energy of collar at A
(TA)g = Wc ha
= 5(10+12) = 110lb.in
For potential energy of collar at C
(Tc)g = Wc*hc
= 5*10= 50lb.in
For potential energy of spring at A
(TA)s = 1/2 *k*óa²
= (1/2)*(2)(10²) = 100lb.in
Potential energy of collqr at c
(Tc)s = 1/2* 2* 3.62²= 13.12
Kinetoc energy of collar at C =
Vc = (1/2)(Wc/g)Vc²
= (1/2)(5/32.2*12) Vc²
= 0.00647Vc² lb.in
From conservation of mechanical energy
Ea= Ec
(Ta)g + (Ta)s = (Tc)g + (Tc)s + Vc
= 110 +100 = 50+13.1+0.00647Vc²
Vc= 150.68 in/s => 12.55ft/s
b) using free body diagram (attached)
let's find the angle made by spring at point C
We have:
∅ =
![tan^-^1[12/10]](https://img.qammunity.org/2021/formulas/engineering/college/uxilwmiyjn91svtpex7v2d507pbyhu2xtn.png)
= 50.19
Therefore, apply force on the horizontal direction, we have:
£fn =

f_4 sin∅ - N = (Wc/g)(Vc²/12)
= (2*3.62)(sin50.19°) - N = [5/(32.2*12)][12.55²/12]
N = 5.392lb