Question

A toy cannon launches a 46-g golf ball straight up into the air with a kinetic energy of 6.8 J. What must the

ball's velocity be as it leaves the cannon?

A. 0.54 m/s

B. 0.79 m/s

C. 17 m/s

D. 80 m/s

Answer 1

Answer:17 m/s

Explanation: To find velocity, use the equation for kinetic energy
`KE = 1/2mv^(2)`. This becomes
`v=√(2KE/m)` .Replace the variables with the values and get
`v = √(2*6.8/0.046) = 17 m/s`.

Answer 2

Answer : The correct option is, (C) 17 m/s

Explanation :

Formula used :

`K.E=(1)/(2)mv^2`

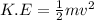
where,

K.E = kinetic energy = 6.8 J

m = mass of object = 46 g = 0.046 kg (1 kg = 1000 g)

v = velocity

Now put all the given values in the above formula, we get:

`K.E=(1)/(2)mv^2`

`6.8J=(1)/(2)* 0.046kg* v^2`

`6.8kg.m^2/s^2=(1)/(2)* 0.046kg* v^2`

`v=17.19m/s\approx 17m/s`

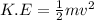
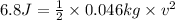
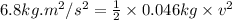
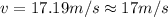
Therefore, the ball's velocity be as it leaves the cannon is, 17 m/s