Final answer:
The investment will be worth approximately $126.98 after 11 years.
Step-by-step explanation:
To find the value of the investment after 11 years, we need to determine the common ratio for the geometric sequence. We know that the initial investment of $4 is worth $144 after 5 years. Using the formula for compound interest, we can set up the equation:
$4
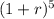 = $144
= $144
Solving for r, we get r ≈ 1.6494. Now we can use this common ratio to find the value after 11 years:
$4
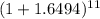 ≈ $126.98
≈ $126.98
Therefore, the investment will be worth approximately $126.98 after 11 years.