the final speed in m/s of the 10.0 kg is 2.53 m/s .
Explanation:
Here we have , A 10.0 kg and a 2.0 kg cart approach each other on a horizontal friction less air track. Their total kinetic energy before collision is 96 ). Assume their collision is elastic. We need to find What is the final speed in m/s of the 10.0 kg mass if that of the 2.0 kg mass is 8.0 m/s . Let's find out:
We know that in an elastic collision :
⇒ Total kinetic energy before collision = Total kinetic energy after collision
⇒

⇒

⇒

⇒
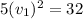
⇒

⇒
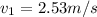
Therefore , the final speed in m/s of the 10.0 kg is 2.53 m/s .